
Calculus
Visual introductions to the core ideas of derivatives, integrals, limits and more

The Essence of CalculusAn overview of what calculus is all about, with an emphasis on making it seem like something students could discover for themselves. The central example is that of rediscovering the formula for a circle's area, and how this is an isolated instance of the fundamental theorem of calculusChapter 1Apr 28, 2017
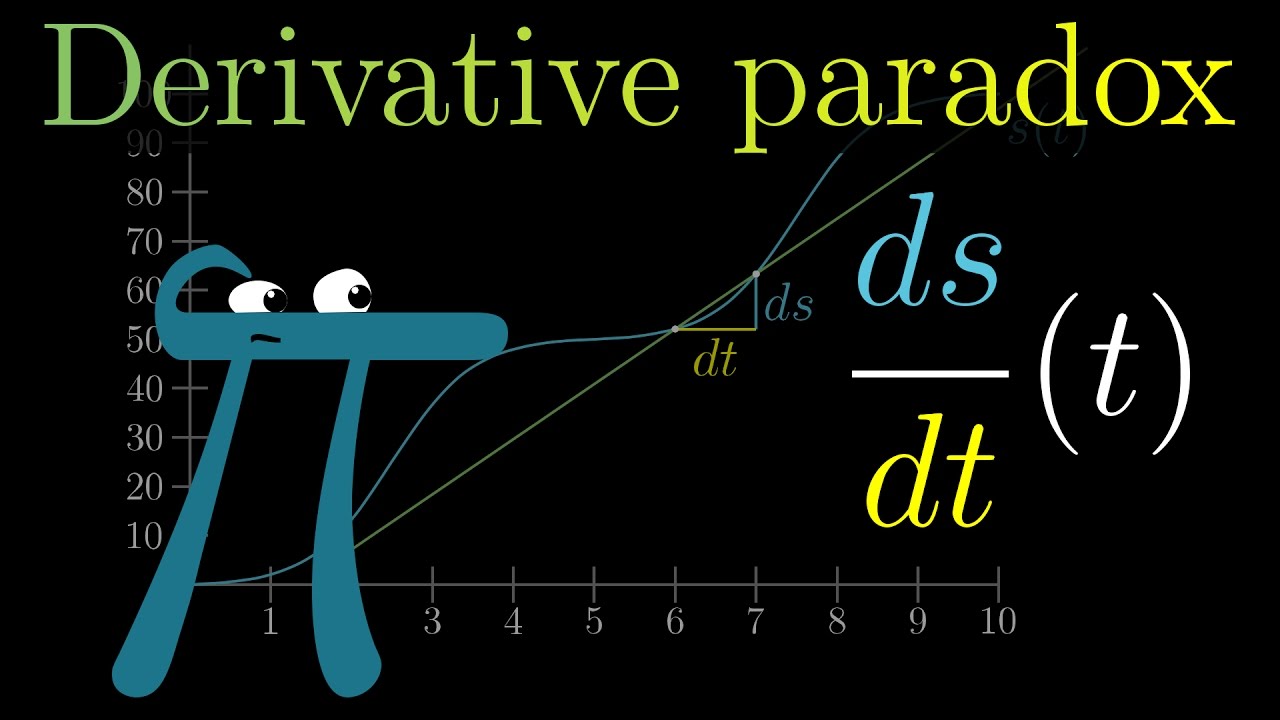
The paradox of the derivativeAn introduction to what a derivative is, and how it formalizes an otherwise paradoxical idea.Chapter 2Apr 29, 2017
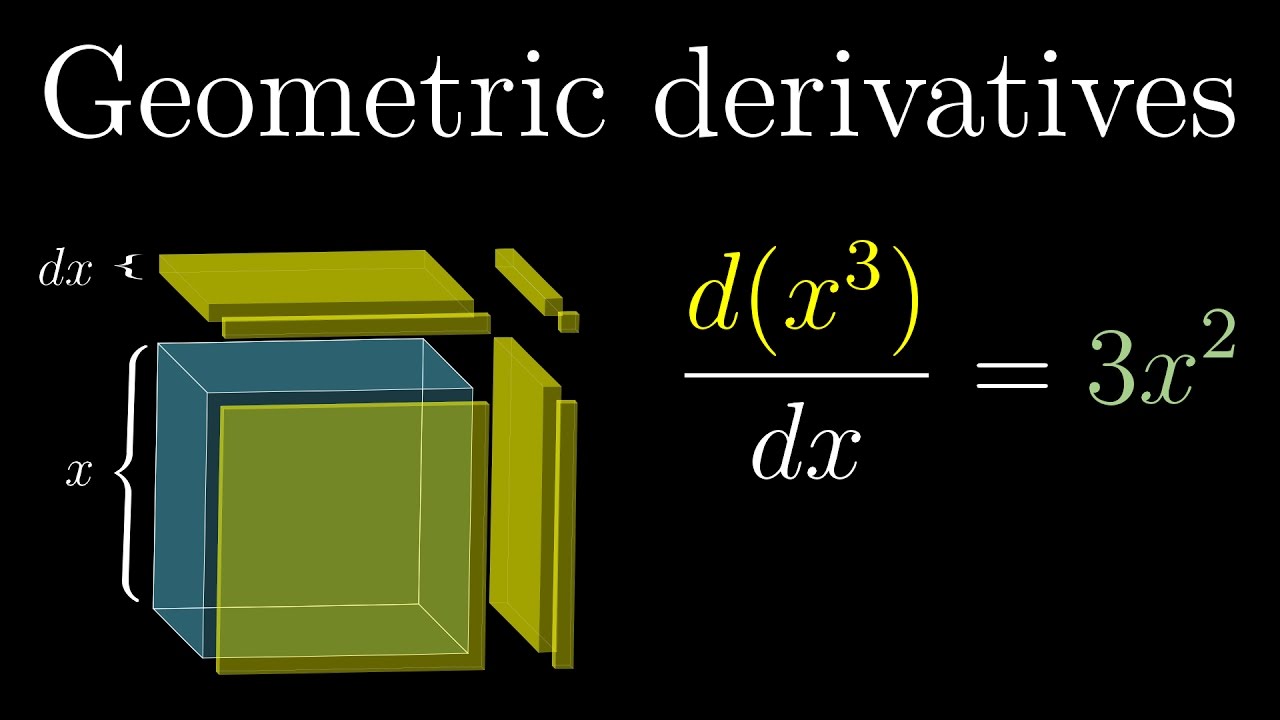
Power Rule through geometryIntroduction to the derivatives of polynomial terms thought about geometrically and intuitively. The goal is for these formulas to feel like something the student could have discovered, rather than something to be memorized.Chapter 3Apr 30, 2017
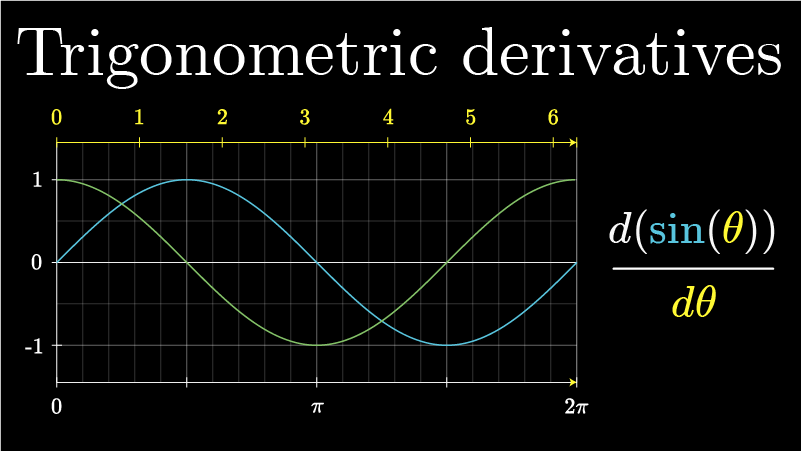
Trig Derivatives through geometryIntroduction to the derivatives trigonometric functions thought about geometrically and intuitively.Chapter 4Apr 30, 2017
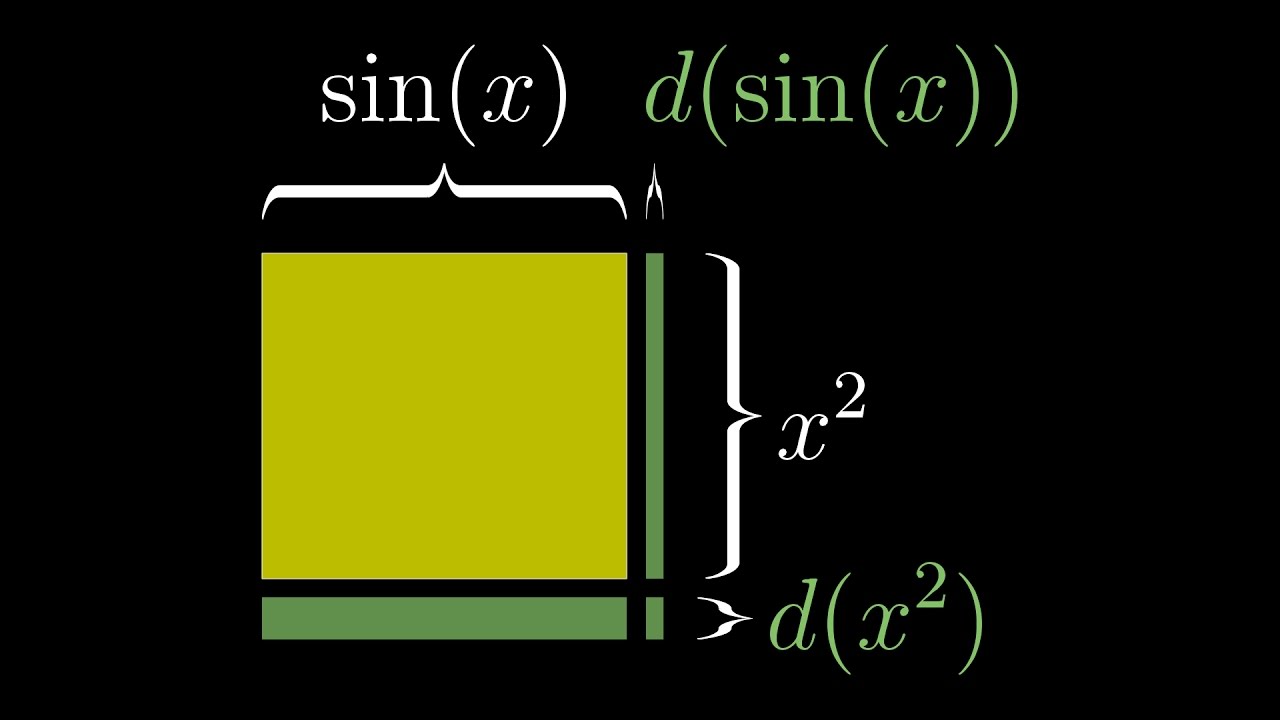
Visualizing the chain rule and product ruleThe product rule and chain rule in calculus can feel like they were pulled out of thin air, but is there an intuitive way to think about them?Chapter 5May 1, 2017
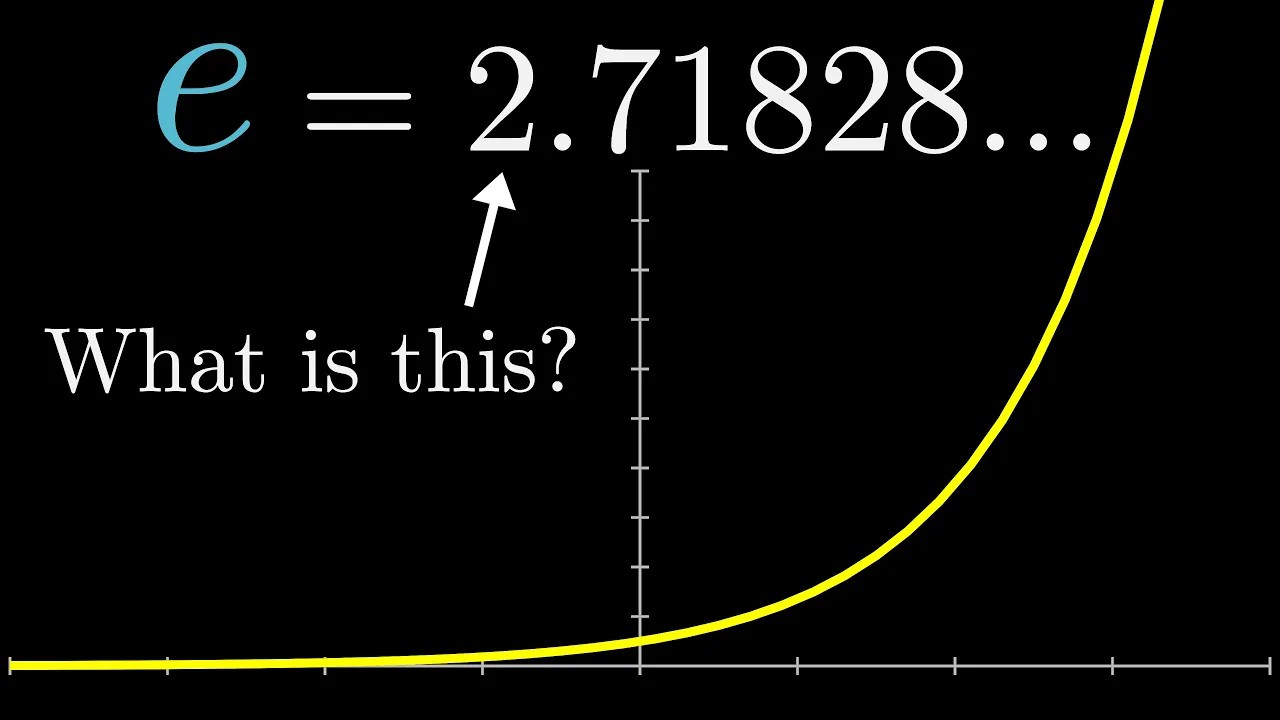
What's so special about Euler's number e?What is the derivative of a^x? Why is e^x its own derivative? This video shows how to think about the rule for differentiating exponential functions.Chapter 6May 2, 2017

Implicit differentiation, what's going on here?How to think about implicit differentiation in terms of functions with multiple inputs, and tiny nudges to those inputs.Chapter 7May 3, 2017
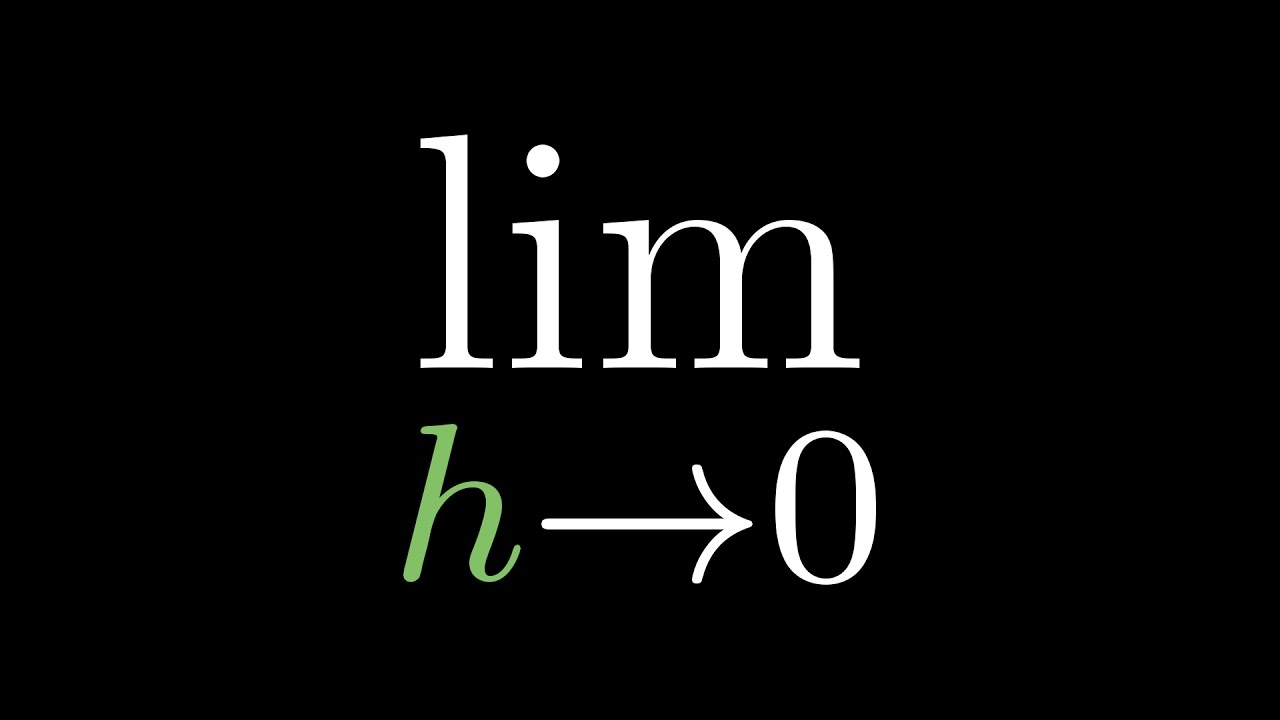
Limits and the definition of derivativesWhat are limits? How are they defined? How are they used to define the derivative?Chapter 8May 4, 2017
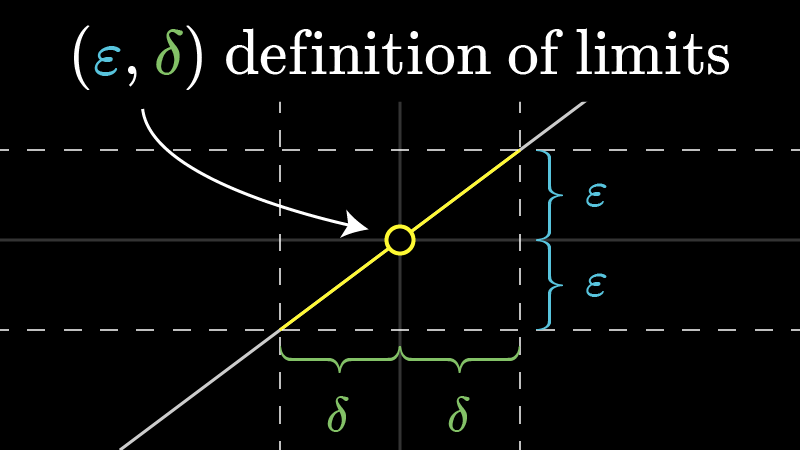
(ε, δ) "epsilon delta" definitions of limitsHow does (ε, δ) "epsilon delta" help us formalize what exactly it means for one value to approach another?Chapter 9May 4, 2017
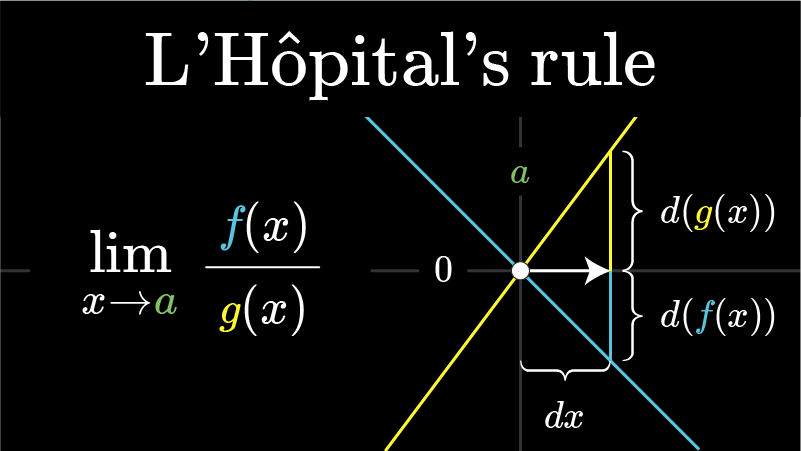
L'Hôpital's ruleWhat is L'Hopital's rule and how does it help us evaluate limits?Chapter 10May 4, 2017

Integration and the fundamental theorem of calculusWhat is integration? Why is it computed as the opposite of differentiation? What is the fundamental theorem of calculus?Chapter 11May 5, 2017
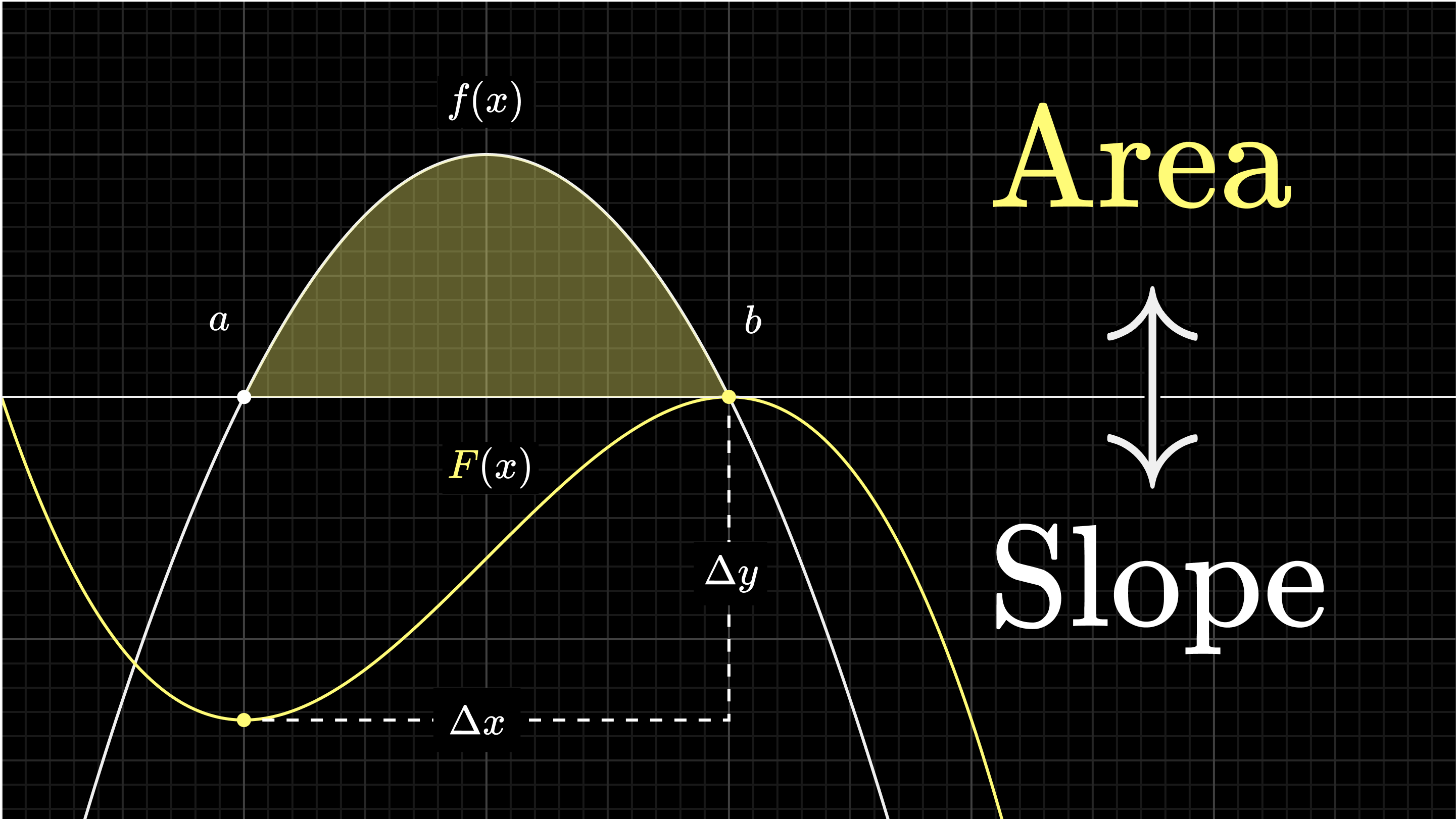
What does area have to do with slope?Derivatives are about slope, and integration is about area. These ideas seem completely different, so why are they inverses?Chapter 12May 6, 2017
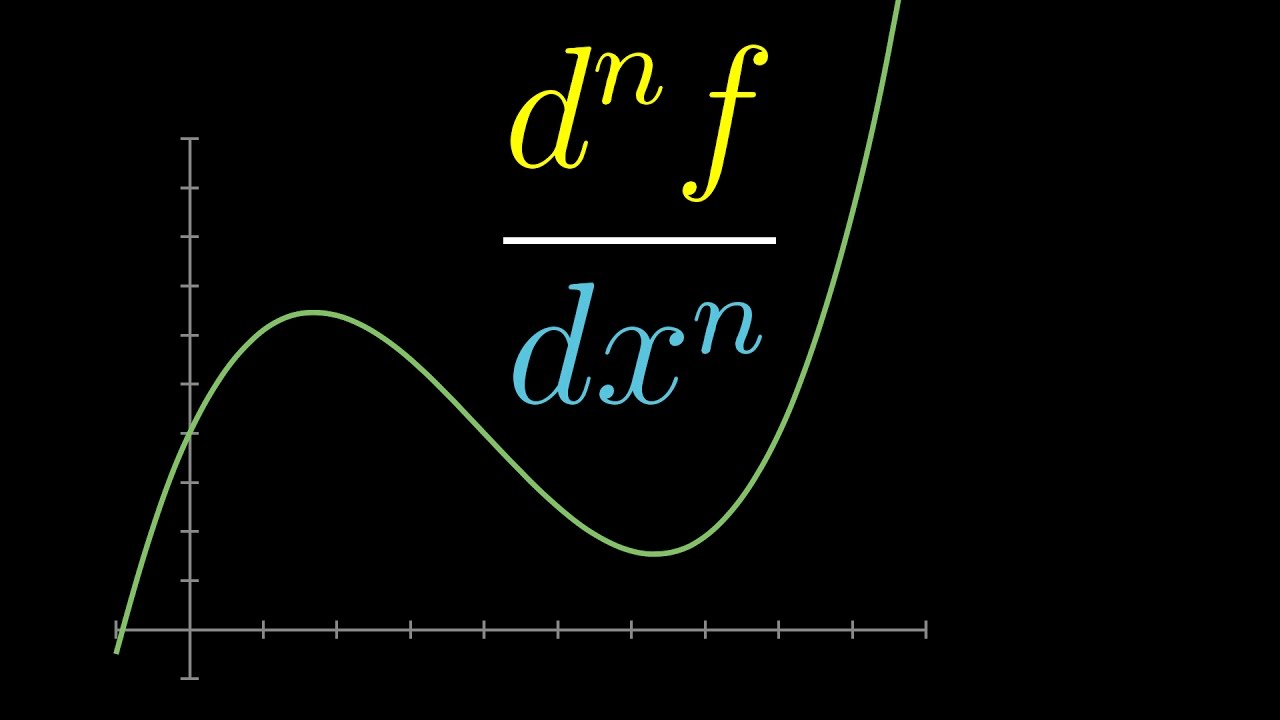
Higher order derivativesWhat is the second derivative? Third derivative? How do you think about these?Chapter 13May 7, 2017
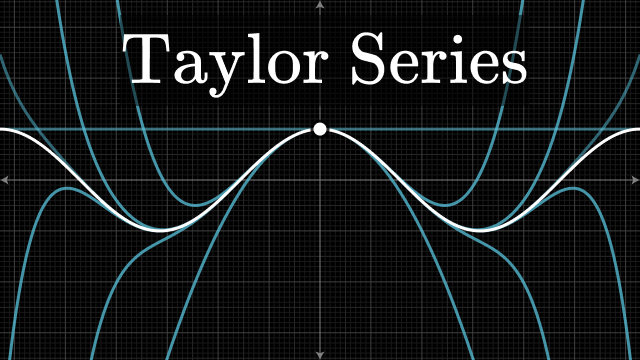
Taylor seriesTaylor series are extremely useful in engineering and math, but what are they? This video shows why they're useful, and how to make sense of the formula.Chapter 14May 7, 2017
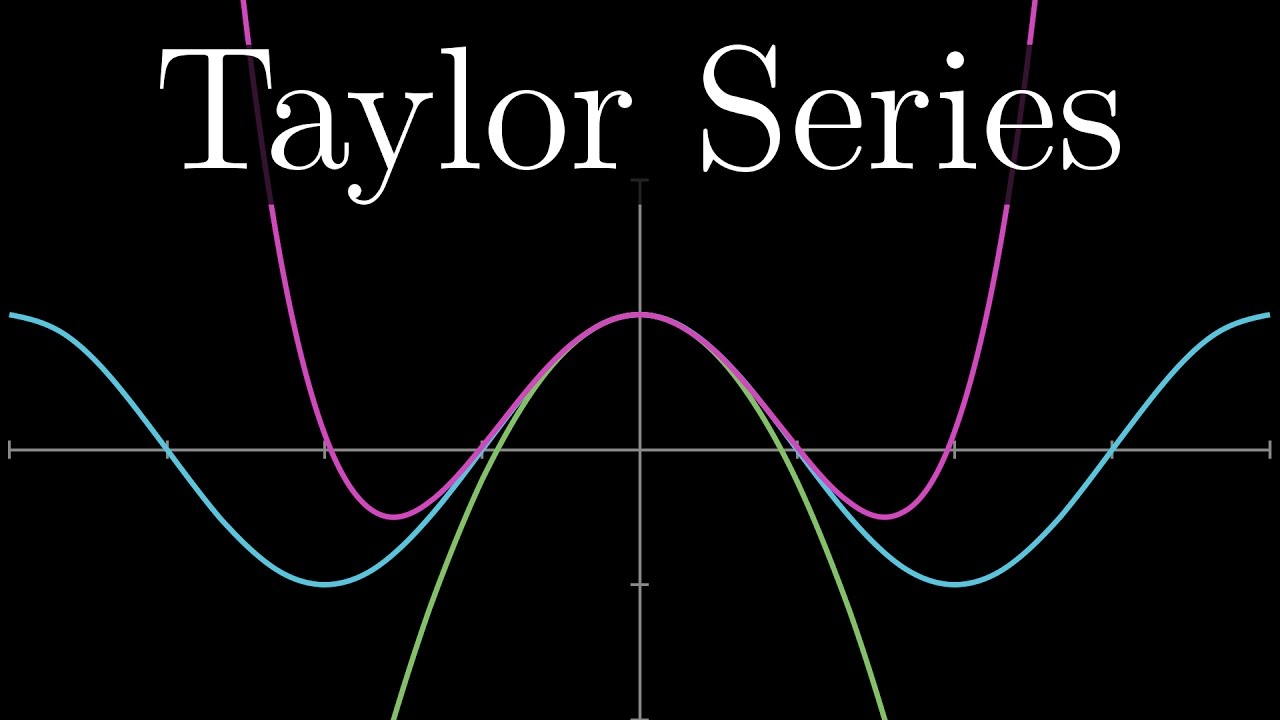
Taylor series (geometric view)A different perspective of Taylor Series that's related to the fundamental theorem of calculus.Chapter 15May 7, 2017
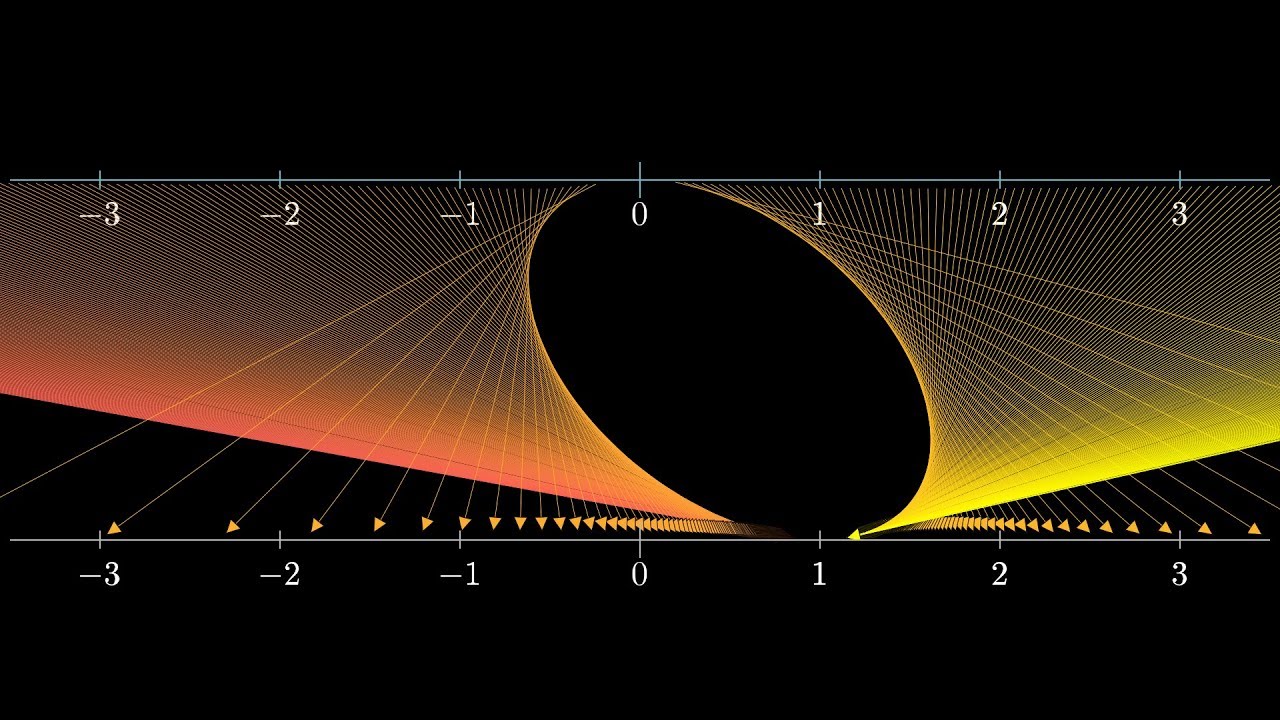
The other way to visualize derivativesA visual for derivatives which generalizes more nicely to topics beyond calculus. Thinking of a function as a transformation, the derivative measure how much that function locally stretches or squishes a given region.Chapter 16May 19, 2018